The Notes
-
1) I've seen this, while dealing with a real analyis problem. It can be very usefull proving statements.
$$\forall x,y\in[0,\infty),\quad \sqrt{|x-y|}\ge |\sqrt x -\sqrt y|$$
$$\circ--------------------------------\circ$$
2) Again, in real analysis.
$$\forall f\neq0,\quad \left(f+\dfrac1f -2\right)=\left(f-1 \right)\left(1-\dfrac1f \right)$$
$$\circ--------------------------------\circ$$
3)$$\forall x_1,x_2\in[0,\infty),\quad|sin^2x_1-sin^2x_2|\le 2|x_1-x_2|$$
$$\circ--------------------------------\circ$$
4)$$ \text{For } \;\; k \in \{0, \cdots, m\}\;\;\text{ we have}$$
$$ A_k = \lim_{x\to-k} \frac{x+k}{x(x+1)\cdots(x+m)} = \frac{(-1)^k}{k!(m-k)!} = \frac{(-1)^k}{m!}\binom{m}{k}. $$$$\Rightarrow$$$$ \frac{1}{x(x+1)\cdots(x+m)} = \frac{1}{m!} \sum_{k=0}^{m} \binom{m}{k} \frac{(-1)^k}{x+k} $$
$$\circ--------------------------------\circ$$
5)$$\text{Let}\;\;\;\; S_n \;\;\;\; \text{be permutation group and}\;\; A_n \;\;\;\;\text{alternating group which is normal subgroup of}\;\; S_n.$$
$$S_n=A_n\cup (12)A_n\;\;\;\;\text{or}\;\;\;\; S_n=A_n\cup t\circ A_n$$ is true for any transposition t.
$$\circ--------------------------------\circ$$
6) Additional to the 5), $$|A_n|=|(12)A_n|=|t\circ A_n|=n!/2$$
$$\circ--------------------------------\circ$$
7)$$ \text{If one wants to find center of} \;\;\;\; GL_2(\mathbb R), \;\;\;\;\text{the trick that one can use is considering invertible matrix}\;\;\;\; \left[ \begin{matrix} 1 & 1 \\ 0 & 1 \end{matrix}\right] \\ \text{since every element in center should commute with it we can explore the general charastics of center of}\;\;\;\; G \;\;\;\;, Z(G)\\ \text{which is } Z(G)=\left \{\left[ \begin{matrix} a & 0 \\ 0 & a \end{matrix}\right] \big | a\in\mathbb {R^x}\right\}$$
$$\circ--------------------------------\circ$$
8) A way to create isomorphism between complex numbers and certain matrices:
$$ (\star )\left[ \begin{matrix} 0 & -1 \\ 1 & 0 \end{matrix}\right]^2=-\left[ \begin{matrix} 1 & 0 \\ 0 & 1 \end{matrix}\right]\quad \Rightarrow\quad \left[ \begin{matrix} 0 & -1 \\ 1 & 0 \end{matrix}\right]=\sqrt{-\left[ \begin{matrix} 1 & 0 \\ 0 & 1 \end{matrix}\right]}$$
Using $(\star)$ we have following: $$\left[ \begin{matrix} a & -b \\ b & a \end{matrix}\right]=a\left[ \begin{matrix} 1 & 0 \\ 0 & 1 \end{matrix}\right]+b\sqrt{-\left[ \begin{matrix} 1 & 0 \\ 0 & 1 \end{matrix}\right]}\approx a+b\sqrt{-1}=a+bi$$
$$\circ--------------------------------\circ$$
9)A very usefull periodic identity. (i)
$f:\mathbb R\to \mathbb R$ be continuous and periodic with period $T>0$ then:
$$(\forall a\in \mathbb R),\quad\displaystyle\int_a^{a+T}f(x)dx=\displaystyle\int^T_0f(x)dx$$
$$\circ--------------------------------\circ$$
10)A very usefull periodic identity. (ii)
$f:\mathbb R\to \mathbb R$ be continuous and periodic with period $T>0$ then:
$$(\forall a<b \in \mathbb R),\quad\lim_{n\to \infty}\displaystyle\int_a^b f(nx)dx=\dfrac{b-a}{T}\displaystyle\int^T_0f(x)dx$$
$$\circ--------------------------------\circ$$
11) "Every group is isomorphic to a group of permutations." A motivation can be given as following.
Considering the cayley table of a group we obtain a permutation for $g_i,g_j\in G$ that $g_i g_j \in G=\{g_1,g_2,...,g_n,...\}$
$$\circ--------------------------------\circ$$
12) $$\arctan\left(\frac{a_1}{a_2}\right)+\arctan\left(\frac{b_1}{b_2}\right)=\arctan\left(\frac{a_1b_2+
a_2b_1}{a_2b_2-a_1b_1}\right)$$
$$\circ--------------------------------\circ$$
13.i) $$\arctan\tanh(n+1)-\arctan\tanh(n-1) = \arctan\left(\frac{\tanh(n+1)-\tanh(n-1)}{1+\tanh(n-1)\tanh(n+1)}\right)=\\=\arctan\left(\frac{\sinh(2)}{\cosh(2n)}\right)$$
$$\circ--------------------------------\circ$$
13.ii) Like in 13.i we have $$\arctan\left(\frac{\sinh(1)}{\cosh(2n)}\right) = \arctan\tanh\left(n+\frac{1}{2}\right)-\arctan\tanh\left(n-\frac{1}{2}\right)$$
$$\circ--------------------------------\circ$$
14) More generally (from m.s.e.) $$\displaystyle\sum_j \arctan x_j= \arctan \frac{\sum_j x_j - \sum_{j,k,\ell} x_j x_k x_\ell + \sum\text{products of five} - \sum\text{products of seven} + \cdots }{1 - \sum_{j,k} x_j x_k + \sum\text{products of four} - \sum\text{products of six} + \cdots}$$
$$\circ--------------------------------\circ$$
15)
$\forall n\in \mathbb N^+$
$$\left\lfloor\sqrt{n^2+2n}\right\rfloor=n$$
$$$$
Generally if $a,b\in\mathbb R^+$ with $a<b$ then $\lfloor a\rfloor \le \lfloor b\rfloor$
$$$$
Proof:
Assume $a,b\in\mathbb R^+$ with $a < b $ but $ \lfloor a \rfloor> \lfloor b \rfloor $
$$$$
$\lfloor a\rfloor > \lfloor b\rfloor$ implies $ \lfloor a\rfloor \ge \lfloor b\rfloor+1$ and
naturally from $a<b$ we got $ \lfloor a\rfloor \le a < b < \lfloor b\rfloor +1$ and using $ \lfloor a\rfloor \ge \lfloor b\rfloor+1$
$$$$
we got : $$ \lfloor b\rfloor+1 \le \lfloor a\rfloor \le a < b < \lfloor b\rfloor +1$$
but $$\lfloor b\rfloor +1<\lfloor b\rfloor +1$$ is absurd.
$$\circ--------------------------------\circ$$
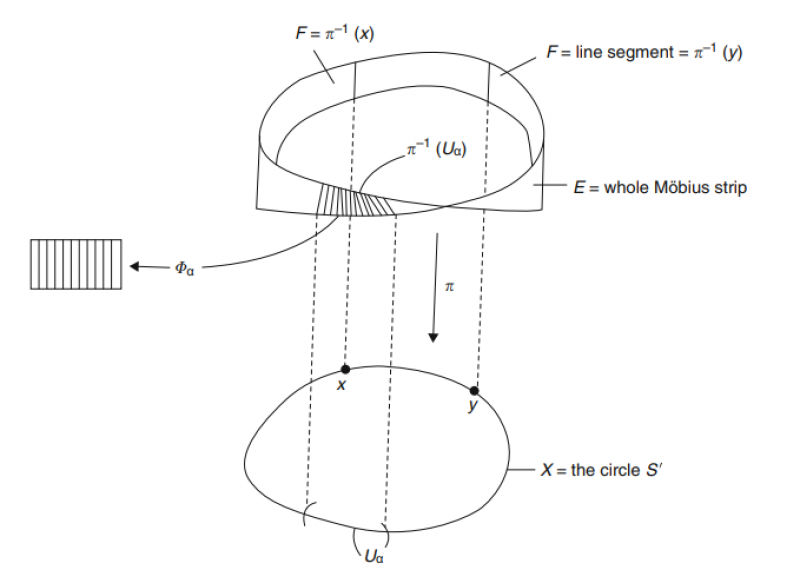
incredible thanks
I’ve been browsing online more than 2 hours
today, yet I never found any interesting article
like yours. It is pretty worth enough for me. Personally, if all website owners and bloggers
made good content as you did, the web will be much more useful than ever before. http://alternatif188bet.com
thanks.
Needed that figure, I really enjoyed to read that math.
is it about your graduate project? nice work tahnk you
işime yaradı mı? evet.. saolun hocam..